
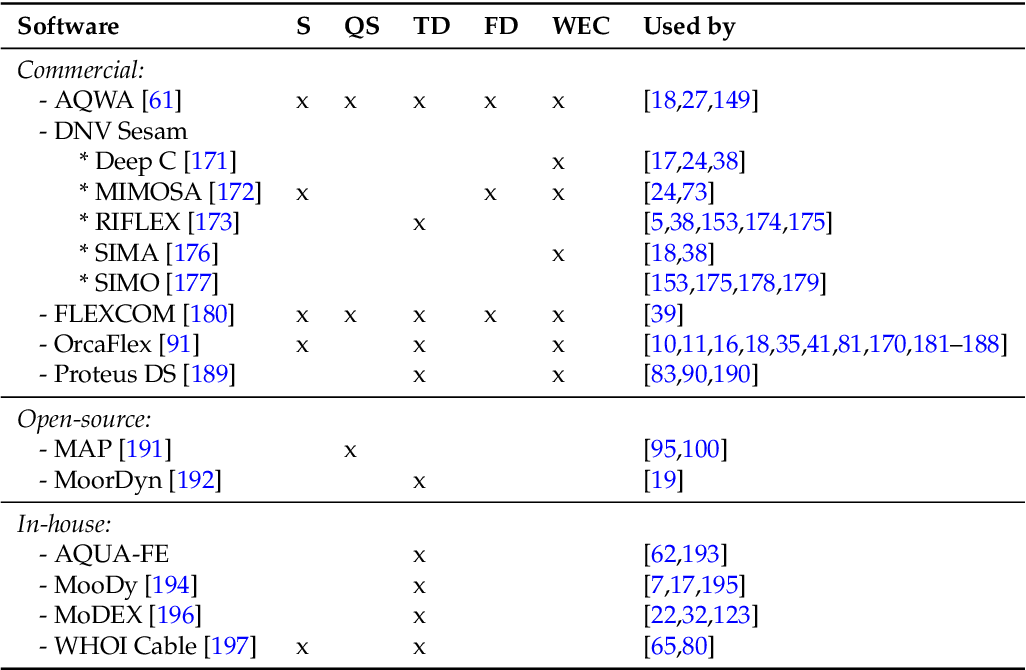
Inclusion of Structural Flexibility in Design Load Analysis for Wave Energy Converters: Preprint A controller controls operation of the PTO, causing the PTO to act as a motor to widen a wave frequency spectrum that is usable to generate electrical energy. Robinett, III, Rush D.Ī wave energy converter (WEC) is described, the WEC including a power take off (PTO) that converts relative motion of bodies of the WEC into electrical energy.

When efficient body wave to surface wave conversion mechanisms are present in the shallow crustal layers, such as soft sediment layers, the scattered surface waves dominate the seismic coda at even early arrival times for shallow sources and at later arrival times for deeper events. The observed common decay trends on seismic coda of local earthquake recordings particular at long lapse times suggest that perhaps later seismic codas are dominated by scattered surface waves. Since surface waves are 2-D propagating waves, their scattered energies should in theory follow a common decay curve. At long distances from the source, scattered surface waves dominate scattered body waves at surface stations. The results show that scattered wave energy can be well approximated by body-wave to body wave scattering at earlier arrival times and short distances. The scattering equations are solved numerically by first discretizing the model at regular grids and then solving the linear integral equations iteratively. (1991), Zeng (1993) and Sato (1994a) on multiple isotropic-scattering, and may shed new insight into the seismic coda wave interpretation. The work is an extended study of Zeng et al. Based on the radiative transfer theory, I developed a new set of scattered wave energy equations by including scattered surface waves and body wave to surface wave scattering conversions. In this paper, I revisit Aki's original idea of the role of scattered surface waves in the seismic coda. One of the many important contributions that Aki has made to seismology pertains to the origin of coda waves (Aki, 1969 Aki and Chouet, 1975). Scattered surface wave energy in the seismic coda The viscous damping on the submerged body should be as small as possible for a given mass in both regular and irregular waves. In regular waves, it is found that the mass of the submerged body should be designed with an optimal value in order to achieve the maximum absorption power for the given floating buoy. It is found that the absorption power of the two- body system can be significantly higher than that of the single body system with the same floating buoy in both regular and irregular waves. A case study is conducted to investigate the influence of the submerged body on the absorption power of a two- body system subjected to suboptimal and optimal design under regular and irregular wave excitations. The physical insight of the optimal design is to have one of the damped natural frequencies of the two body system the same as, or as close as possible to, the excitation frequency. The suboptimal and optimal designs for a two- body system are proposed based on the closed form solution. The closed form solution of the maximum absorption power and corresponding power take-off parameters are obtained. A linearized model in the frequency domain is adopted to study the dynamics of such a two- body system with consideration of both the viscous damping and the hydrodynamic damping. The energy is extracted through the relative motion between the floating and submerged bodies. On The Dynamics and Design of a Two- body Wave Energy ConverterĪ two- body wave energy converter oscillating in heave is studied in this paper. For the subcritical velocities, the law governing the motion of the body and the ratio of the kinetic energy variation to the energy supplied to the body are determined. The process of the body acceleration along a beam of the aforementioned type is investigated. The equations that describe the motion of a body along a string and along a beam corresponding to the Bernoulli-Euler model are considered as examples. An equation describing the longitudinal motion of a body along an arbitrary guide is derived from the laws governing the energy and momentum variations for the case when the incident wave generates a single reflected wave. The motion of a body along an elastic guide under the effect of an incident wave is considered. Transformation of Elastic Wave Energy to the Energy of Motion of Bodies


 0 kommentar(er)
0 kommentar(er)
